box muller joint distribution • Inverse transform sampling• Marsaglia polar method, similar transform to Box–Muller, which uses Cartesian coordinates, instead of polar coordinates See more I want to install a MOCA network, but I'm confused where the POE filter goes within the cable junction box that's on the outside of my house. There are several pics below that show the box, what the wires look like when I open the .
0 · proof of box muller
1 · how to calculate box muller
2 · box-muller method exercise
3 · box muller wikipedia
4 · box muller method pdf
5 · box muller method formula
6 · box muller maths
7 · box muller formula
It bends easily at first, slowly resisting more and more until it reaches a point and pushes back to return to its original shape. The noises resultant from that pent up kinetic energy may vary, but it's not unlike a bow on a string or a mallet on a drum.
The Box–Muller transform, by George Edward Pelham Box and Mervin Edgar Muller, is a random number sampling method for generating pairs of independent, standard, normally distributed (zero expectation, unit variance) random numbers, given a source of uniformly distributed random numbers. The . See moreSuppose U1 and U2 are independent samples chosen from the uniform distribution on the unit interval (0, 1). Let See moreThe polar method differs from the basic method in that it is a type of rejection sampling. It discards some generated random numbers, but can be faster than the basic method . See more
• Inverse transform sampling• Marsaglia polar method, similar transform to Box–Muller, which uses Cartesian coordinates, instead of polar coordinates See more
• Weisstein, Eric W. "Box-Muller Transformation". MathWorld.• How to Convert a Uniform Distribution to a Gaussian Distribution (C Code) See moreThe polar form was first proposed by J. Bell and then modified by R. Knop. While several different versions of the polar method have been described, the version of R. Knop will be . See moreC++The standard Box–Muller transform generates values from the standard normal distribution (i.e. standard normal deviates) with mean 0 and standard deviation 1. The implementation below in standard See more
Exercise (Box–Muller method): Let U and V be independent random variables that are uniformly distributed on [0, 1]. Define X: = √− 2log(U)cos(2πV) and Y: = √− .1 Box Muller It would be nice to get a standard normal from a standard uniform by inverting the distribution function, but there is no closed form formula for this distribution function N(x) = P(X . A transformation which transforms from a two-dimensional continuous uniform distribution to a two-dimensional bivariate normal distribution (or complex normal distribution).Transformation Methods: Box-Muller Algorithm I Proposition. If R2 ˘Exp(1 2) and ˘U[0;2ˇ] are independent then X= Rcos , Y = Rsin are independent with X˘N(0;1); Y ˘N(0;1): Proof: We .
The Box–Muller transform is a pseudo-random number sampling method for generating pairs of independent, standard, normally distributed (zero expectation, unit variance) random numbers, given a source of uniformly .
proof of box muller

One can show that the density of R is fR(r) = re − r2 / 2 and the density of Θ is fΘ(θ) = θ / 2π. So the joint density of R and Θ is fR, Θ(r, θ) = re − r2 / 2 / 2π. Box-Muller transform is a method used to produce a normal distribution. Imagine two independent distributions of X, Y ~N (0,1) plotted in the Cartesian field. Then, we need some distance.
types of sheet metal seams
Here we want to show that the Box-Muller method generates a pair of independent standard Gaussian random variables. But I don't understand why we use the determinant? For me when you have two indep. Recalling now that the joint pdf of two independent uniforms is the unit square, we have that the joint distribution of the transformatons is. f(z0, z1) = 1 2πexp{− 1 2(z20 + z21)}, − .The Box–Muller transform, by George Edward Pelham Box and Mervin Edgar Muller, [1] is a random number sampling method for generating pairs of independent, standard, normally distributed (zero expectation, unit variance) random numbers, given a source of uniformly distributed random numbers.
Exercise (Box–Muller method): Let U and V be independent random variables that are uniformly distributed on [0, 1]. Define X: = √− 2log(U)cos(2πV) and Y: = √− 2log(U)sin(2πV). Show that X and Y are independent and N0, 1 -distributed.1 Box Muller It would be nice to get a standard normal from a standard uniform by inverting the distribution function, but there is no closed form formula for this distribution function N(x) = P(X < x) = √1 2π R x −∞ e−x02/2dx0. The Box Muller method is a brilliant trick to overcome this by producing two independent standard normals A transformation which transforms from a two-dimensional continuous uniform distribution to a two-dimensional bivariate normal distribution (or complex normal distribution).
how to calculate box muller
Transformation Methods: Box-Muller Algorithm I Proposition. If R2 ˘Exp(1 2) and ˘U[0;2ˇ] are independent then X= Rcos , Y = Rsin are independent with X˘N(0;1); Y ˘N(0;1): Proof: We have f R2; (r 2 ) = 1 2 exp r2=2 1 2ˇ and therefore we are interested in f X;Y(x;y) = f R2; (r 2(x;y); (x;y)) 2 det @(r; ) @(x;y) where 2 det @(r; ) @(x;y) @r . The Box–Muller transform is a pseudo-random number sampling method for generating pairs of independent, standard, normally distributed (zero expectation, unit variance) random numbers, given a source of uniformly distributed random numbers.
One can show that the density of R is fR(r) = re − r2 / 2 and the density of Θ is fΘ(θ) = θ / 2π. So the joint density of R and Θ is fR, Θ(r, θ) = re − r2 / 2 / 2π. Box-Muller transform is a method used to produce a normal distribution. Imagine two independent distributions of X, Y ~N (0,1) plotted in the Cartesian field. Then, we need some distance.
Here we want to show that the Box-Muller method generates a pair of independent standard Gaussian random variables. But I don't understand why we use the determinant? For me when you have two indep.
Recalling now that the joint pdf of two independent uniforms is the unit square, we have that the joint distribution of the transformatons is. f(z0, z1) = 1 2πexp{− 1 2(z20 + z21)}, − ∞
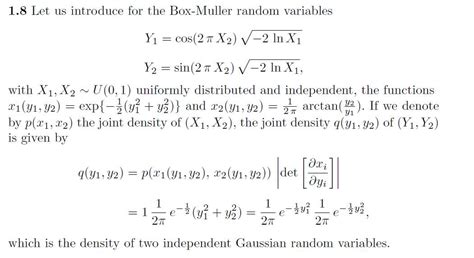
Exercise (Box–Muller method): Let U and V be independent random variables that are uniformly distributed on [0, 1]. Define X: = √− 2log(U)cos(2πV) and Y: = √− 2log(U)sin(2πV). Show that X and Y are independent and N0, 1 -distributed.1 Box Muller It would be nice to get a standard normal from a standard uniform by inverting the distribution function, but there is no closed form formula for this distribution function N(x) = P(X < x) = √1 2π R x −∞ e−x02/2dx0. The Box Muller method is a brilliant trick to overcome this by producing two independent standard normals
A transformation which transforms from a two-dimensional continuous uniform distribution to a two-dimensional bivariate normal distribution (or complex normal distribution).
box-muller method exercise

Transformation Methods: Box-Muller Algorithm I Proposition. If R2 ˘Exp(1 2) and ˘U[0;2ˇ] are independent then X= Rcos , Y = Rsin are independent with X˘N(0;1); Y ˘N(0;1): Proof: We have f R2; (r 2 ) = 1 2 exp r2=2 1 2ˇ and therefore we are interested in f X;Y(x;y) = f R2; (r 2(x;y); (x;y)) 2 det @(r; ) @(x;y) where 2 det @(r; ) @(x;y) @r . The Box–Muller transform is a pseudo-random number sampling method for generating pairs of independent, standard, normally distributed (zero expectation, unit variance) random numbers, given a source of uniformly distributed random numbers.

One can show that the density of R is fR(r) = re − r2 / 2 and the density of Θ is fΘ(θ) = θ / 2π. So the joint density of R and Θ is fR, Θ(r, θ) = re − r2 / 2 / 2π. Box-Muller transform is a method used to produce a normal distribution. Imagine two independent distributions of X, Y ~N (0,1) plotted in the Cartesian field. Then, we need some distance.
Here we want to show that the Box-Muller method generates a pair of independent standard Gaussian random variables. But I don't understand why we use the determinant? For me when you have two indep.
types of cnc router machines
type of junction box
Whether it's tweaking your handlebar reach, height or length for improved comfort and control, or crafting unique footpegs for your custom motorbike, we’ve got you covered. Our custom CNC motorcycle parts are designed and manufactured in the UK from top-grade billet aluminium, guaranteeing top-notch quality and swift delivery.
box muller joint distribution|box muller formula